| | Welcome on MOOC-invitation |
|
| New! | An invitation to practice origami | map | Practical work Geometric constructions |
| Back MOOC1 | Journey 3 | Sequence 1 | <--- page 2 | page 3 | page 4---> |
 |
Create any convex hexagon |
| This is a very simple exercise in appearance, but one that requires attention. The document to download gives a diagram to create any convex hexagon. This is to make 6 consecutive folds, so that the final end of the last fold joins the initial end of the first fold. Try some variations: - fold a convex (almost) regular hexagon (the 6 sides are equal), - fold a convex hexagon whose first three sides are larger than the last three, - fold a convex hexagon of which one side out of two is larger than the adjacent sides, - Generalize the process by folding a heptagon, a hexagon, a dodecagon, a convex n-gon. One hundred penny question: Can you fold a non-convex hexagon, for example looking as a star, without using cutouts? Treat your folds so that the folds are beautiful to see. |
1 document(s) to download
| mlhexaqq.pdf | Créer un hexagone convexe quelconque, 1 page |
7 photo(s)
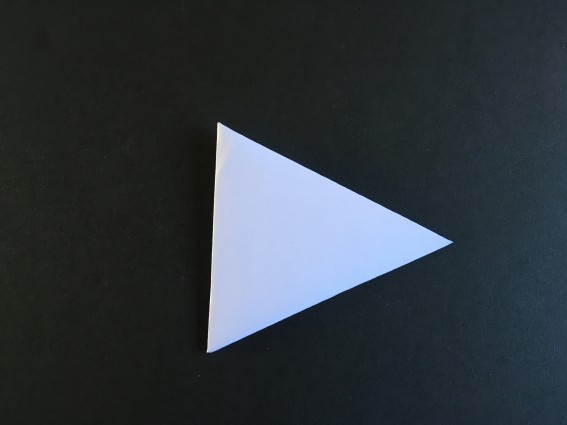triangle | 
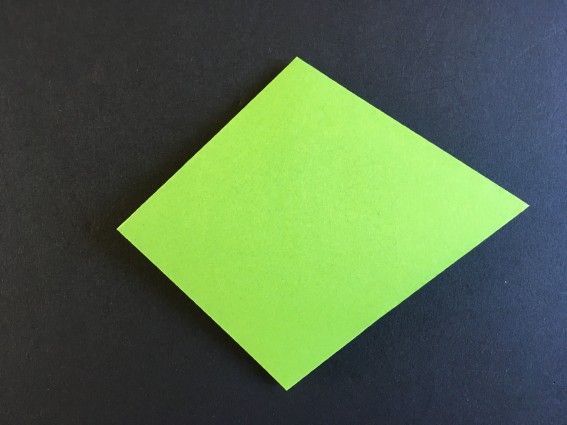quadrilateral | 
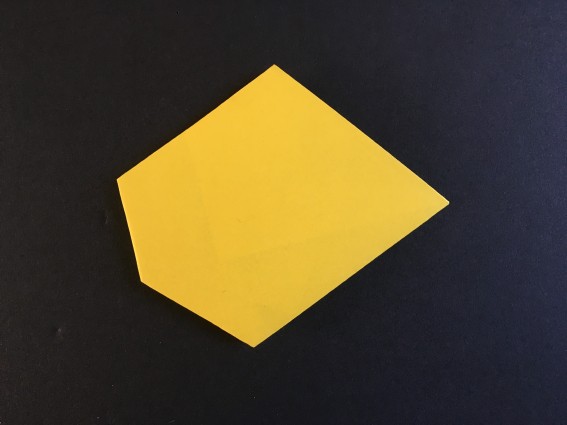pentagon |
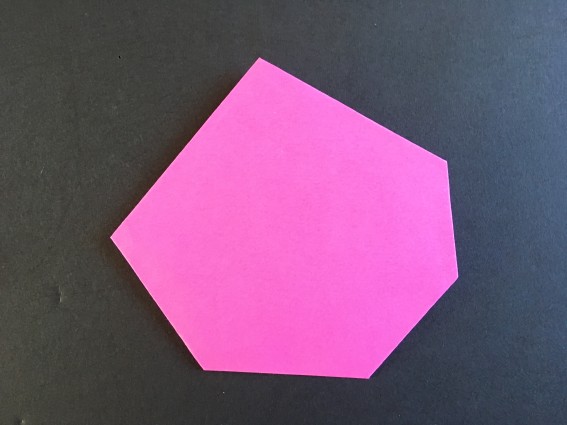hexagon | 
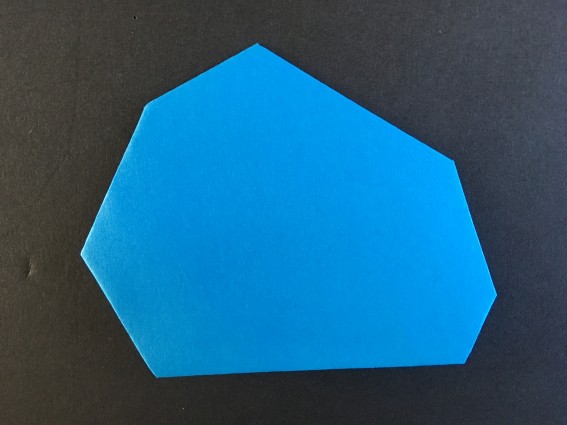heptagon | 
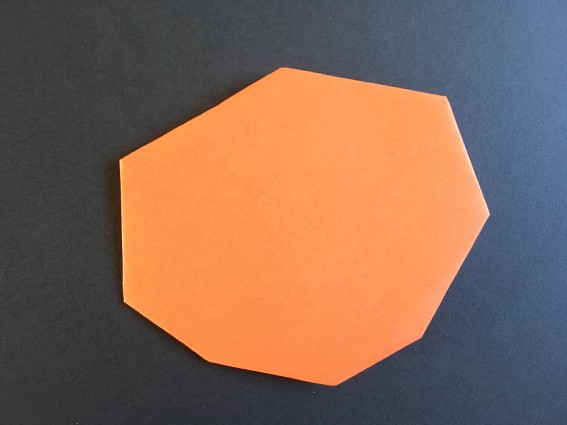octogon |
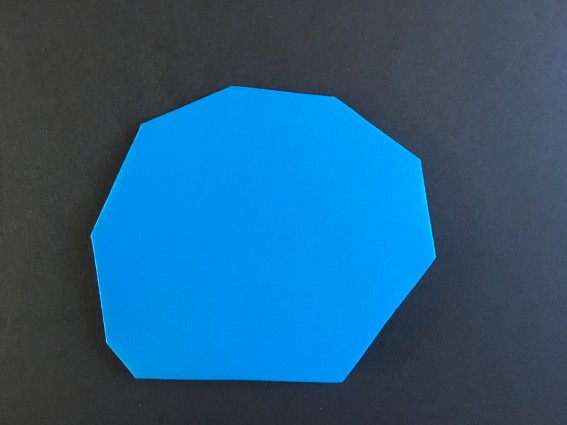nonagon |